Back to a more educational point of view now following
the last post I wrote. Today will be a look back, potentially in more detail,
at the concept of indifference analysis. I'll try and start at the very basics
and work my way through the subject - if you feel I've left anything out do not
hesitate to let me know in a comment and I'll try and go over it for you. This post is long, I won't hide that fact. Make full use of the search bar to the left of this to make sure this contains what you're looking for. Even better still, use Ctrl + F and search for keywords. That could save you some time!
Indifference analysis is a basic concept in economics
which looks at consumers preferences for two goods. It is an "exactly what
it says on the tin" topic - we are looking at combinations of these two
goods that the consumer would feel indifferent about. The definition of
'Indifferent' from dictionary.com, by the way, is "having no bias, prejudice, or preference; impartial; disinterested." So,
rephrasing it into Layman's terms: we are looking at combinations of these two
goods that the consumer would feel equally as happy, content, etc with. This will
become evident later on in the analysis.
The very first step in the analysis will be to construct an indifference curve. This is done below.
Here we have an indifference curve. This is modelling
different combinations of Good A and Good B that the consumer would feel
indifferent about. Anywhere along this curve the consumer will be feeling the
same level of satisfaction. Indifference curve slope downwards - that is a
general rule. Why? I hear you ask. Well, it's due to the diminishing marginal
rate of substitution. This piece of jargon essentially means the rate at which
we would swap Good A(Y) for Good B(X) while remaining equally as satisfied. It
looks as follows:
If we used some figures as an example, let's say that the consumer is indifferent between 25 of Good A and 5 of Good B and is also indifferent between 20 of Good A and 6 of Good B. Right. So, the top of the equation would be (20 - 25) and the bottom of the equation would be (6 - 5). That leaves us with -5 as the answer. So, between the points (25,5) and (20,6) on a diagram the slope would be -5. We can focus in on the negative sign here, this shows why the curve is always downwards sloping. We have to give up some of Good A to get more of Good B. Keeping up? Good.
An
indifference curve alone tells us very little, an indifference map on the other
hand tells us a lot more. An indifference map is a series of indifference
curves showing which combinations of two goods give different levels of
satisfaction.
If you think of the map as a mountain, starting from the
bottom left corner and working diagonally to the right and up - the higher up
the mountain we go the more satisfied the consumer. At any point on I4 the
consumer is more satisfied than at any point on I2 for example. The question
that creeps up a lot regarding indifference curves is "Could they ever
cross?" In short, the answer is no. This can be proved via contradiction.
Consider the three points here: a, b and c. From the
analysis we've just done we can say that a is indifferent to b. We can also say
that a is indifferent to c. So, b 'should' be indifferent to c. But b has more
of Good A for the same amount of Good B than c does, and therefore point b
would be preferred. b and c aren't indifferent, and therefore indifference
curves cannot cross. Bosh!
Now, for a consumer to make a decision about what to consume
we need more information than just the indifference curves. We need information
on prices and incomes. This is where the budget line enters. I fear I'm stating
the obvious here, but I'll have to continue: the budget line is how much of the
two goods the consumer can afford. So, it'll have the following formula, which
reads 'Price of Good A times the quantity of Good A plus the price of Good B
times the quantity of Good B equals the consumers income:
If
Good A was £2, Good B was £1 and the
consumer's income was £30 then we'd have an equation to work out: 2A + B = 30.
Now we have our equation we can plot the budget line on a graph.
It is literally as simple
as that for the budget line. All we need is the price of the two goods and the
consumer's income and we can work out the quantities of each good they can
purchase. While on the topic of the budget line I think I'll mention what
happens to the line when prices and incomes change. If just one price changes
then the budget line will swing in or out, pivoting around a point. For
example, if the price of Good B fell, then we'd see the line swing out to the
right, pivoting around the point on the Y axis. It would swing out because a
fall in price of Good B means we can afford more of them. A rise in price
causes a swing in. A change in the total budget or consumer's income means a
shift in the whole budget line parallel to the current one. A rise in the
budget shifts the curve out to the right, a fall in the budget shifts it in to
the left. If both incomes and prices rise by the same percentage, or fall by
the same percentage for that matter, we see no change in the budget line.
Moving
swiftly on, we're ready to combine the indifference map and the budget line.
This can give us the consumer's optimal consumption point. Utility or
satisfaction for the consumer is maximised at the point of tangency between the
budget constraint and the indifference map, as is highlighted in the graph
below.
The slope of the indifference curve is the marginal rate
of substitution and the slope of the budget constraint is (minus) the relative
price of Good A and Good B. Therefore, where these meet, the consumer chooses
optimally when MRS = Price of Good B / Price of Good A.
Another piece of jargon you may need to learn is the
'Price-Consumption curve'. This is a curve derived from the changing price of
one of the goods. From this curve we can create a demand curve for that good.
Clever stuff.
Now, to derive the demand curve from it. We make one
alteration to the diagram above, we make the Y axis 'Expenditure on all other
goods' instead of just Good A. Then, we follow the points of tangent down and
onto a new diagram. On the Y axis of the below diagram we list the prices,
which come from dividing the Budget by the quantity of Good B when expenditure
on all other goods is 0. Match these prices up to the lines we've just drawn
down and Bob's your uncle - a demand line. In words it sounds confusing, take a
look at the diagram below and then re-read this until sense is made.
I hope that makes sense - reread and study the diagram.
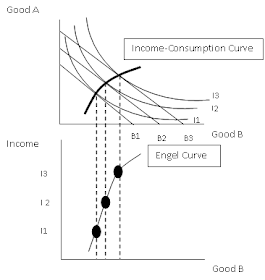
Another 'special' curve we need to be aware of is the
Income-Consumption curve. This tracks the effect a change in income has on our
optimum choices of the two goods. The slope of this curve tells us about the desirability
of Good A and Good B as incomes rise. In general, the curve will look something
like this:
There is a special case where the shape of this curve
bends the other way. This is when one of the goods is an inferior good - I
trust we all know what that means. A higher budget will mean less is demanded
and therefore the income consumption curve will bend back on itself.
Bravo to those of you that have made it this far and
hello to those that skipped straight to this section. Neither of you will be
judged... honest. Finally, we are going to look at the Engel Curve. An Engel
Curve shows how the demand for a good changes as income changes. We use the
Income-Consumption curve and track it down onto a new diagram below.
If incomes increase and this leads to a demand increase
for the good then we are looking at a normal good. If demand decreases it is an
inferior good. The final, more peculiar outcome, is in the case of a giffen
good. Giffen good prices rise when demand rises, odd - but they do exist.
By gosh, I think we might be finished. I said
'potentially' more in depth at the beginning - I think that word can be
scrapped. If you're still unsure of anything Indifference Analysis related then
drop me a comment and I'll be happy to try and help you out if I can. Thank you
for reading, have a good day!
Sam.










No comments:
Post a Comment